Geoboard
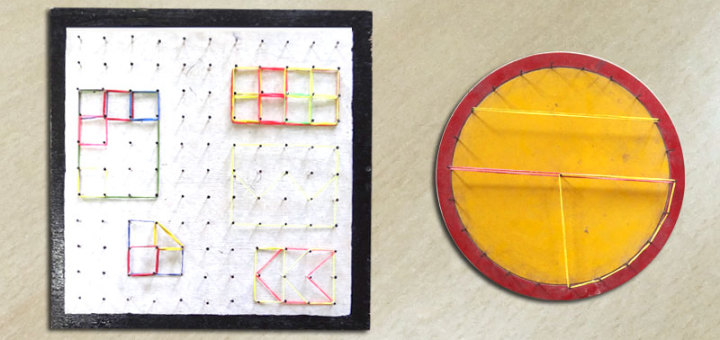
Material Required: Square board “10 x 10”of wood or acrylic, nails or pegs, super-glue if acrylic board is used.
A Geoboard is a very useful device for introducing children to important topics in school geometry. It consists of an array of nails or pegs which are placed at equal distances on a square acrylic board making up a grid. The baseboard is made from wood or acrylic. Drill holes at equal distances forming a square grid. It is convenient to place the pegs at gaps of 1cm. Nails can also be used, but the heads must be small. Acrylic boards with holes drilled in them are good for use on an overhead projector. Graph paper can also be placed below an acrylic board which is useful in estimating areas. The pegs or nails can be glued onto the acrylic board.
Polygonal figures can be made on the Geoboard by stretching rubber bands across the pegs. Some of the activities that can be performed are
- Property of similar figures: Take two rubber bands and form the triangles one within the other. Are they similar? Why or why not?
- On the Geoboard it is very easy to find areas of figures by completing rectangles. Hence it is easy to verify theorems about areas. For example, with two rubber bands the theorem, “the area of a triangle on the same base and between the same parallels is half that of the parallelogram” can be verified.
- Another activity on the Geoboard is to construct right angle triangles such that none of the sides of the triangle is aligned along a row or a column. How many such triangles can be constructed? What is the relation between the sides of these triangles?
- An interesting activity to do with the Geoboard is to discover the Pick’s theorem. The steps for this activity are described briefly:
- Make different polygonal shapes on the Geoboard. Can you determine the areas of these polygonal figures?
- There is a simple formula for obtaining the area of a polygonal figure on the Geoboard. It is not difficult to derive this formula. Try to!
- First begin with a simple figure that is easy — the unit square. What happens to the square as you deform one of its four sides, keeping its other three sides intact? Make a change in one of the sides of the square and describe the result by answering the questions below:
* What is happening to the number of boundary points? Is it increasing or decreasing or remaining the same?
* What is happening to the number of points in the interior the polygon? Is it increasing? Are any of the boundary points becoming interior points?
* Prepare a table for each step as you change one side of the square. Similarly start with a rectangle and observe what happens. Can you arrive at a general rule for the area of a polygon?
The general rule for areas of polygonal figures is given by the formula,
A = + I – 1.
A — is the area of the figure,
B — number of boundary points,
I — number of interior points



play adopt me on roblox give me a uni and