Missing Square
Missing area 1
Material Required: Card paper, graph paper(optional), pencil or pen, cutter or scissors.
The missing square and missing line activities take everyone by surprise. But there is a perfectly clear explanation for the mysterious missing square and missing line.
Procedure:
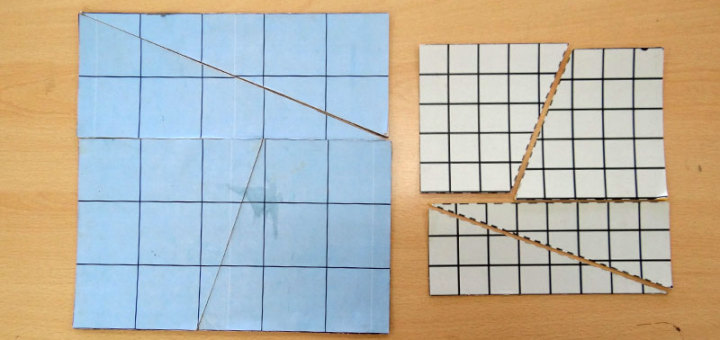
To make the missing square puzzle, cut a paper into “5 by 5” square. Form 25 smaller squares by drawing lines for every inch. Now cut this square along the dotted line shown in the figure to get four pieces. These four pieces on rearranging into a rectangle give us only 8 x 3 = 24 squares, leaving us surprised as one square is missing from the original piece. The same activity can be performed on a “8 x 8” square and cutting it into four pieces as above. On rearranging the pieces into a rectangle we get 13 x 5 = 65 squares, this time one more than the original.
The reason for this increase or decrease in the number of square is simple. In each case when we cut the square into pieces and re-assemble them to form the rectangle, the small squares that are obtained are not perfect squares, but are slightly enlarged or compressed. Sometimes the edges do not match. In order to obtain perfect squares, the pieces have to overlap slightly. The total area of overlap will be equal to the area of one square, thus bringing down the number of squares from say 25 to 24. Alternatively gaps may have to be left along the junction lines to get perfect squares. In this case area is added and the total increase in area is equal to the area of one square. The number of squares then increases say from 64 to 65 in the 8 x 8 square.
Missing Square 2
Material Required:Card paper, graph paper(optional), pencil or pen, cutter or scissors.
Missing square puzzle contains a right angle triangle with base 13 units and height 5 unit which is formed by four components
1. A right angle triangle with dimensions 8 units 3 units
2. A right angle triangle with dimension 5 units
\times
2 units
3. A L shaped figure with 7 sq. Units
4. A L shaped figure with 8 sq. Units
Rearranging these 4 pieces to form a new triangle with the same dimension as shown in the figure.
Both the triangles form 13 unit
\times
5 unit right angle triangle, but the second arrangement has a missing square in it. Where did the square go?
It can be noticed that the area of both the triangles and the combined area of the components are different.
Area of the components: Area of piece 1 =
\frac{1}{2}\times
8
\times
3 =12 sq.units
Area of piece 2=
\frac{1}{2}\times
5
\times
2 = 5 sq.units
Area of piece 3 = 7 sq.units
Area of piece 4 = 8 sq. Units
Total area of components is 32 sq. Units
Calculated area =
\frac{1}{2}\times
13
\times
5 = 32.5 sq. Units
So calculated area and combined area doesn’t match. Why it is so?
Notice the hypotenuse of the piece 1 and piece 2. hypotenuse of piece 1 has a slope of
\frac{3}{8}
where as the hypotenuse of piece 2 has a slope of
\frac{2}{5}
. so when combining these two lines don’t constitute a single line for both the triangles. The combined hypotenuse in both the triangles are actually bent.
When overlapping both these triangles, overlaying hypotenuses results a very thin parallelogram with area of exactly one square, the same area “ missing” from the rearranged figure.